Gravity and the Equivalence Principle of General Relativity

Already in ancient times, it was a well known fact that all objects (regardless of their mass) fall with the same acceleration due to gravity. This was later measured more accurately by Galileo, Newton and others (see here for more ).
In terms of Newton's law of gravity, this circumstance is expressed by the fact that in the equation

(1) G^.m_g^.M/r² = m_i^.a

the 'inertial mass' m_i is equal to the 'gravitational mass' m_g i.e. m_i =m_g=m (note that this equality is however only the result of adjusting (as a matter of convention) the gravitational constant G suitably; experiments can indeed only reveal that the two must be proportional). So the acceleration due to gravity

(2) G^.M/r² = a

is thus independent of the mass of the accelerated object but depends only on the mass of the body providing the gravity. Einstein now suggested that this circumstance would justify the assumption that gravitational acceleration is in all respects equivalent to the observer being accelerated instead (in the opposite direction) in the absence of gravity:

"we [...] assume the complete physical equivalence of a gravitational field and a corresponding acceleration of the reference system." (Einstein 1907).

Einstein did actually not formulate this principle in mathematical terms, but rather tried to justify it through thought experiments and practical analogies. He was arguing along the lines: if one drops an object subject to gravity in a lab resting on the earth's surface, one observes the same as if one would drop an object in a lab in space accelerated in the direction of the ceiling (e.g. by a rocket motor). However, even though the apparent resulting force is the same, it is obvious that this does not imply a complete physical equivalence of the situation, because in the former case the object is accelerated (due to force of gravity) whilst the lab is resting, but in the latter case the lab is accelerated (due the force provided by the rocket motor) whilst the object is resting. So contrary to Einstein's superficial interpretation of the situation, there is a profound difference here with regard to the physics involved. Relativists always stress (see for instance (this page from the 'Einstein-Online' Website) that the equivalence principle only holds strictly locally because of the fact that the gravitational field is in practice not homogeneous; with this restriction however it is claimed that it is an exact principle i.e. if the lab is scaled down to sufficiently small dimensions it should be impossible for an experimenter to determine by a local measurement alone whether the apparent gravity is due to the gravitational attraction of a mass or due to an acceleration of his lab. However, it is obvious that even then one can in general distinguish the two situations: it is just required that one exerts a force on the floor or ceiling of the lab from the inside; this will evidently change the apparent gravity in a lab in space but not in a lab on the earth (as the mass of the latter is too high to result in a measurable change of acceleration).

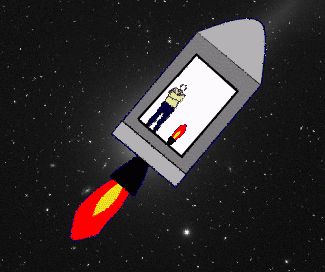Space Lab 'Gravity'

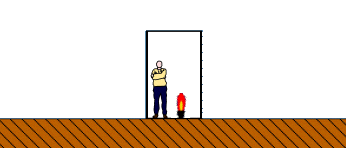Ground Lab Gravity

In these illustrations it is suggested that the experimenter fires a small rocket engine fixed to the floor inside the lab. Until the exhaust fumes reach the ceiling, this will impart an acceleration which counteracts the acceleration provided by the external rocket motor and thus reduce the gravity accordingly. However, the same experiment in the ground lab will not produce a noticeable change, as the mass of the earth (to which the lab is effectively fixed) is much too high for this. Of course, one does not need to fire a rocket engine inside the lab to figure out whether one is in space or on the earth, but in principle almost anything one does in the lab will produce a difference, even just lifting an arm or leg. Also for instance, despite the identical apparent gravity, the period of a pendulum inside the two labs will be different as we are dealing with a two body problem and the period is affected by the mass of the lab.

This demonstrates that it is in fact impossible to make a correct interpretation of a local measurement without taking the global physical situation into account. Ignoring the latter and replacing it by one that is 'equivalent' in a certain respect can in general not give a correct description of physical reality. In this case, this means that forces due to gravity have to be considered separately from other forces

The conceptual flaw of this gravity/acceleration equivalence principle is not only evident from a suitable modification of the usual thought experiments (as illustrated above), but also if one considers it from a logical/mathematical point of view, because Eq.(2), with the usual understanding of physical equations, can be read as: "a particle at a distance r from a mass M experiences an acceleration a=G^.M/r² ". In logical notation, one could write therefore (M,r)=>a for this causal relationship between the physical parameters M and r on the one hand and the resultant kinematical acceleration 'a'. A complete equivalence between the two sides in this relationship would now mean that one would instead have (M,r)<=>a i.e. one could also read the equation backwards as "any acceleration 'a' causes a gravitational field". Einstein indeed explicitly states this in chapter XX of his book Relativity: The Special and General Theory (see the last paragraph, where he claims that the braking of a train would produce a gravitational field). First of all, this inversion is actually not possible, for the simple reason that the left hand side of (M,r)<=>a is given by two parameters (M,r) but the right hand side only by one (a), so the inversion would not be uniquely physically determined (a given acceleration 'a' could be associated with an infinite number of pairs (M,r)). Apart from this, a causal loop would be set up which should result in the gravitational field produced by the acceleration accelerating the object further (and so on), so we would have a case of a 'gravitational catastrophe', which obviously is not observed in reality. From a logical point of view, it is therefore non-sensical to postulate an equivalence between a physical cause and its effect.

Of course, the reason for these paradoxes and inconsistencies resulting from the assumption of a complete equivalence of gravity and acceleration is that in general the acceleration of a mass m is not given by Eq.(2) but

(2a) G^.M/r² + F_non-grav/m = a ,

so the acceleration is not only determined by gravity but also by other (non-gravitational) forces F_non-grav. And as the latter are in general not proportional to the mass m, the equivalence principle is therefore not applicable to them, and therefore not for the total acceleration either.

In this sense, the General Theory of Relativity must be considered as a conceptually flawed construct as it is very much based on the equivalence principle as outlined above (this is ignoring even the fact that incorporates the also flawed results of Special Relativity; see for instance the page Mathematical Flaws in Einstein's 'On the Electrodynamics of Moving Bodies').

Gravity and the Equivalence Principle of General Relativity

Conceptual Flaws in Einstein's Theory of Gravity