Hooke's Law states that the elongation (strain) of elastic materials is proportional to the applied force. This is primarily a purely empirical macroscopic law which holds only up to a certain relative extension of the object, after which the behaviour becomes plastic and eventually the object fractures (see Fig.3 in
Reference1). The problem is how to interprete these macroscopic properties in terms of atomic physics: for instance, the electrostatic force between two charges decreases with distance ~ 1/r
2, yet the stress force for a macroscopic object increases proportionally to its extension. The latter can therefore only be explained by a collective particle behaviour (Hookes law applies for instance also in plasma physics if one considers collective displacements of the electrons from the ions (plasma oscillations)). Within certain limits, the individual molecular bond apparently also exhibits such a 'harmonic oscillator' behaviour (see
Reference2), but as one can see from the molecular potential curve, it is much too strong (by about a factor 100) to account for the observed stress fracture point of materials (which occurs already at a relative extensions (strain) of about 1%). One could try to explain this discrepancy through the circumstance that for larger aggregates of atoms (e.g. crystal lattices) the bond should in principle be much weaker as the electrons are shared by more atoms. As illustrated below, if one has for instance four nuclei forming two individual molecules, there are two electrons forming the bond for each molecule (a), but there is only one electron forming the bonds if all nuclei are arranged in a single aggregate (b)
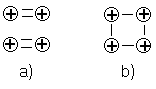
Unfortunately, I could not find any theoretical arguments how this affects the bond in crystal lattices (apparently, it is generally assumed it doesn't), but in my opinion the consequences should be substantial for many materials (the molecular bond is usually relatively weak compared to the electrostatic energies of the individual particles and may not even survive removing half the number of bond electrons). Note that this seems to be also confirmed by the melting point of materials, which indeed corresponds to only roughly 1% of the binding energy of an individual electron.
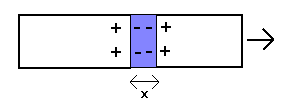
Although a weaker molecular bond would explain the observed breaking stress of materials, it would obviously not account for the elastic behaviour as given by Young's modulus and Hooke's law (for the reason mentioned in the first paragraph). This suggests that the latter might in fact be caused by a different force, and, as already indicated above, it could be related to plasma polarization fields caused by stretching the material: if the positive charges in the material are displaced by a distance x due to an applied force, free electrons will move into the space created and thus create a polarization field (displacement field) which pulls the positive charges back (as illustrated below)
The stress (force per unit area) associated with this is given by the equation
S = 4π.n.e2.x .n2/3 = 4π.n5/3.e2.x ,
where n is the free electron density, e the elementary charge and x the displacement.
Now, Hooke's law holds typically only for strains (relative extensions) of less than 0.3% (see Fig.3 in
Reference1) which requires a stress of the order of S=10
9dyn/cm
2 (i.e. a weight of 1000 kg/cm
2). Assuming the average distance between the nuclei in the material to be 10
-8cm, their corresponding displacement is therefore x=3
.10
-11cm. Inserting these values into the above equation, one finds that the free electron density in the material is n=2
.10
22 cm
-3 (i.e.about 3% of the atom density).
With this interpretation, the maximum possible displacement y of the plasma electrons should depend on their kinetic energy K through the equation
K = 2π.n.e2.y2 .
For thermal electrons of room temperature (300
o K), one finds a value of about y=10
-9cm, i.e. Hooke's law would hold up to a strain of 10%, in contradiction to experiments. The discrepancy could be explained by the neglection of elastic collisions of the plasma electrons which will reduce the displacement to the average collision length
y = 1/(n.σc) ,
where σ
c is the Coulomb collision cross section which for room temperature (300
oK) has a value of about 10
-11cm
2. This yields now a value of 2y=10
-11cm, in good order of magnitude agreement with the observed elastic limit of x=3
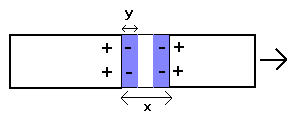
.10
-11cm. For a larger strain than this, the plasma polarization field will fail to bridge the gap between the two sections and the corresponding force will vanish, as schematically depicted below:
The non-linear behaviour in this region (i.e. beyond point 3 in Fig.3 in
Reference1) could then indicate the effect of the collisions in that regime (where the strain is larger than the collision length).
Note: I am aware that other theories exist to explain the relatively small forces needed to achieve stress fracture in materials. These are based on the assumption of stress inhomogeneities in the material due to cracks for instance (e.g. Griffith's theory), but they seem to be rather qualitative and only appear to be able to describe how cracks evolve under stress but not how they develop in the first place. In fact, the crack interpretation suffers in my opinion from a crucial logical flaw: in order to explain the unexpectedly small work required to homogeneously stretch the material to the yield point (elastic limit), the molecular bonding energy would have to be reduced by the cracks to less than 1% of the theoretical value from the outset (i.e. even without any stress applied), which is absolutely inconceivable.
Nevertheless, I am not an expert in material science in general and stress theory in particular and would welcome any clarifying responses in this matter (
Feedback).